Gammafunktion

Eine der wichtigsten nichtelementaren Funktionen ist die sogenannte Gammafunktion (Γ-Funktion):

$\Gamma(x) = \int\limits_0^{\infty} e^{-t} t^{x-1} dt \; \; ,x>0$

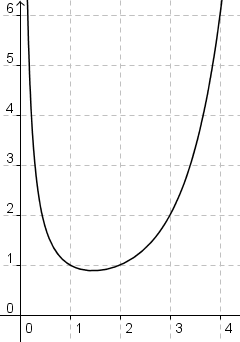
Graph der Gammafunktion, x > 0

Sie ist z.B. Teil der Dichte der wichtigen Chi Quadrat Verteilung.

Die Gammafunktion erfüllt die Beziehung

Γ(x + 1) = x·Γ(x)
Zusammen mit Γ(1) = 1 folgt hieraus

Γ(2) = 1·Γ(1) = 1, Γ(3) = 2·Γ(2) = 2, Γ(4) = 3·Γ(3) = 6, …

Die Gammafunktion nimmt also an den ganzzahligen Stellen n + 1 die Werte n! (n-Fakultät) an, Γ(n + 1) = n!.